How To Calculate Audio System Noise
Product specifications often list the signal-to-noise ratio, sometimes written as “SNR” or “S/N,” but what does it mean? While the math behind SNR is technical, the concept is not, and this value can impact a system’s overall sound quality.
SNR compares the level of a signal to the level of noise. In other words, it compares the ratio between the relevant (wanted) and the irrelevant (unwanted) information. It is most often expressed as a measurement of decibels (dB). Higher numbers generally mean a better sound quality, since there is more useful information (the signal) than there is unwanted data (the noise).
A decibel is a logarithmic ratio in which every 20 dB represents a factor of 10. For example, going from 80dB to 100dB means increasing by 10 times. As an example, if you have an amplifier with a gain of 20dB that means it multiplies its input signal by a factor of 10, one (1) volt on the input becomes 10V on the output.
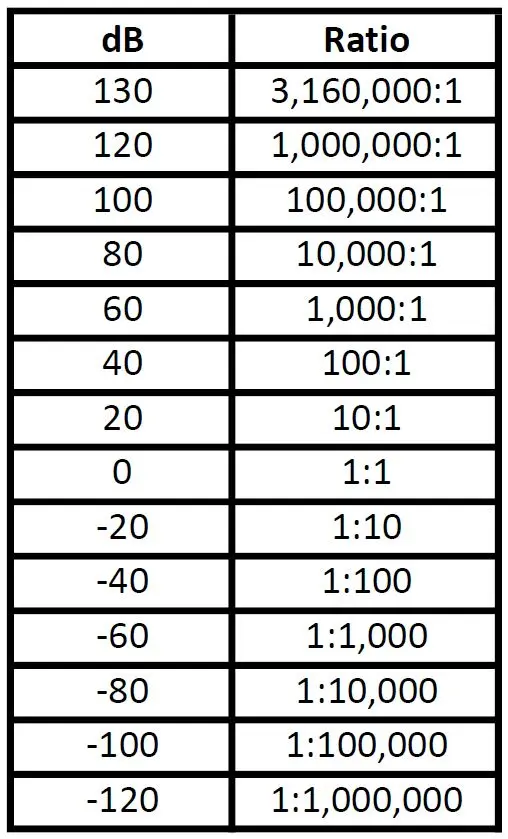
I created the table to the left to show the most common dB ratios.
As an example when you are comparing two products with a signal-to-noise ratio (SNR) of 120 and 100 dB. The 120dB product has 10 times less noise than the one with 100dB spec.
For other dB values and ratios, this is a very good calculator to use: http://www.sengpielaudio.com/calculator-db.htm
Noise is additive that is why it is very important to understand the ratios to help you figure out the total noise in your system.
Continue reading to see how to figure out total system noise.
Total System Noise
Let’s take a look at a typical system that has 3 components, DAC, preamplifier, and power amplifier. For this example, let’s say all 3 components have an SNR of 120db. However, just this information is not enough we also need to know the gain of each stage and the output voltage for each device at which the SNR specification is given. For this example let’s assume the below values:
DAC – Output Voltage 4VRMS
Pre-amp – Output Voltage 10VRMS, Gain -7dB (reasoning for -7dB explained below)
Power Amp – Output Voltage 34.64VRMS (150W into 8Ω), Gain 26dB
To figure out the total system noise, we will start with the DAC and work our way down. The DAC has an output voltage of 4V and an SNR of 120dB. This means that its total output noise is 4uV (0.000004V) or 4V / 1,000,000. Similarly, the pre-amp has a total noise of 10uV and the amplifier has a total noise of 34.64uV.
The gain of the preamp is set to -7dB, the total gain for the system is 19dB such that 4V output from the DAC makes 150W (34.64V) at output of the amplifier.
Now we need to take the gain of each device into account. The gain of any device applies to both signal and noise. In our example, the noise from the DAC is amplified by the pre-amp and then again by the amp. The noise from the DAC, pre-amp, and amplifier are uncorrelated, this means that the addition of the two noise sources has to be done using the square root of the sum of the squares (SRSS) method.
Taking it step-by-step:
Noise at output of pre-amp = 10uV (self-induced)
Noise at output of preamp from DAC = 4uV * -7dB
Noise at output of preamp from DAC = 4uV * 0.45 (-7 dB, use calculator referenced above)
Noise at output of preamp from DAC = 1.8uV
Total noise at output of preamp = sqrt (102 + 1.82) = sqrt(100 + 3.24) = sqrt(103.24) = 10.16uV
Noise at output of power amp = 34.64uV (self-induced)
Noise at output of power amp from pre-amp and DAC = 10.16uV * 26db
Noise at output of power amp from pre-amp and DAC = 10.16uV * 20 (26dB, use calculator referenced above))
Noise at output of power amp from pre-amp and DAC = 203.2uV
Total noise at output of power amp = sqrt (34.642 + 203.22) = sqrt(1200 + 41,290) = sqrt(42,490) = 206.13uV
The system’s SNR can now be figured out. It is the ratio of the total noise to the final output voltage.
SNR = 34.64V / 206.13uV
SNR = 34.64V / 0.00020613V = 168,049
SNR = 104.5dB
Even though you started with 3 components that have very good SNR specs of 120dB (1,000,000:1) the total system SNR ended up being almost 10 times worse at 104.5dB (168,049:1). For reference CD quality audio which is 16-bits has a maximum SNR of 96dB (16 bits x 6dB per bit).
As a comparison let’s take a look at PecanPi Streamer + Starkrimson Mono Amp as a system. In this case, the PecanPi Streamer functions as both DAC and pre-amp
PecanPi Streamer – Output Voltage 5VRMS, SNR 130dB
Starkrimson Monoblock Amp – Output Voltage 34.64VRMS (150W into 8Ω), SNR 121dB, Gain 16.8dB
Taking it step-by-step:
Total noise at output of streamer = 5V / 3,160,000 (130dB) = 1.58uV
Noise at output of amp = 31uV (self-induced)
Noise at output of amp from streamer = 1.58uV * 16.8dB
Noise at output of amp from streamer = 10.92uV
Total noise at output of amp = sqrt (312 + 10.922) = sqrt(961 + 119.2) = sqrt(1080.2) = 32.87uV
The system’s SNR can now be figured out as that is the ratio of the total noise to the final output voltage.
SNR = 34.64V / 32.87uV
SNR = 34.64V / 0.00003287V = 1,053,848
SNR = 120.5dB
Summary
Since the gain of a device multiplies both signal and noise, devices with high gains will have a significant effect to total system performance. Keep the number of gain stages to a minimum as each gain stage adds noise and multiplies the noise from the previous stage.
I have created a calculator for two, three, and four device systems. It is at this link.